Mathematics and science have always been inextricably linked, with advances in one field often catalyzing progress in the other. Throughout history, certain mathematical theorems have emerged as true game changers, revolutionizing our understanding of the universe and enabling unprecedented scientific and technological breakthroughs.
Let us explore five such theorems that have had a profound impact on the course of scientific discovery.
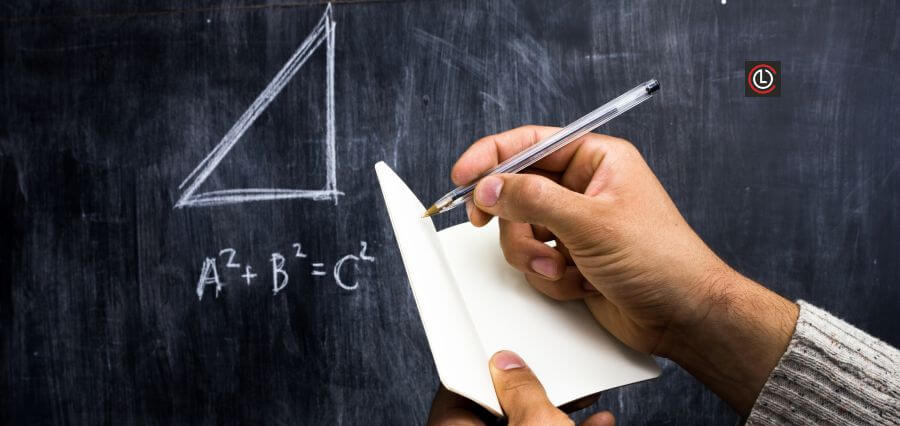
- Pythagorean Theorem
Perhaps the most famous mathematical theorem of all time, the Pythagorean theorem states that in a right-angled triangle, the square of the length of the hypotenuse is equal to the sum of squares of the other two sides. While simple in its formulation, this theorem’s implications are far-reaching.
In physics, the Pythagorean theorem is fundamental to understanding vector addition, a concept crucial in mechanics, electromagnetism, and quantum physics. In astronomy, it helps calculate distances in space. The theorem also forms the basis for more advanced mathematical concepts like trigonometry and complex numbers, which are indispensable in modern science and engineering.
- Calculus (Fundamental Theorem of Calculus)
While not a single theorem, the development of calculus by Newton and Leibniz in the 17th century marked a watershed moment in mathematics and science. The Fundamental Theorem of Calculus, which establishes the relationship between differentiation and integration, is particularly significant.
Calculus provided scientists with powerful tools to describe and analyze change and motion. It became the language of classical physics, allowing for the formulation of Newton’s laws of motion and universal gravitation. Today, calculus is essential in fields ranging from quantum mechanics and relativity to economics and population dynamics.
- Euler’s Identity
Described by physicist Richard Feynman as “the most remarkable formula in mathematics,” Euler’s identity (e^(iπ) + 1 = 0) connects five fundamental mathematical constants in a single, elegant equation. This theorem is more than just a mathematical curiosity; it has profound implications in various scientific fields.
In physics, Euler’s identity is crucial in understanding wave phenomena, from quantum mechanics to signal processing. It plays a vital role in electrical engineering, particularly in the analysis of alternating current circuits. The identity also finds applications in fields as diverse as control theory, fluid dynamics, and even music theory.
- Noether’s Theorem
Emmy Noether’s theorem, published in 1915, establishes a deep connection between symmetries in physics and conservation laws. It states that for every continuous symmetry of the laws of physics, there must exist a corresponding conservation law.
This theorem has been described as one of the most important mathematical theorems ever proven in guiding the development of modern physics. It provides a unifying principle for understanding conservation laws, such as the conservation of energy, momentum, and angular momentum. Noether’s theorem has been instrumental in the development of quantum field theory and particle physics, helping scientists uncover fundamental properties of the universe.
- Shannon’s Information Theory
Claude Shannon’s landmark 1948 paper “A Mathematical Theory of Communication” laid the foundation for information theory. At its core is Shannon’s theorem, which defines the maximum rate at which information can be transmitted over a communication channel.
Shannon’s work revolutionized our understanding of information and communication. It has been crucial in the development of digital communication technologies, from mobile phones to the internet. Beyond communications, information theory has found applications in diverse fields including cryptography, data compression, statistical inference, and even in understanding biological systems like neural networks and DNA.
These five theorems represent just a fraction of the mathematical ideas that have shaped modern science. They demonstrate the incredible power of abstract mathematical thinking in unlocking the secrets of the physical world. As science continues to advance, it’s certain that mathematics will remain an indispensable tool, with new theorems and concepts emerging to drive future discoveries and innovations.
By providing a framework for understanding complex phenomena, quantifying uncertainty, and making precise predictions, these mathematical theorems have truly been game changers in the scientific world. They serve as a testament to the profound and often unexpected ways in which pure mathematics can transform our understanding of the universe and drive technological progress.